Reading Fractions

Slice a pizza, and we get fractions:

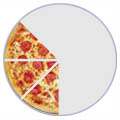1/2	1/4	3/8
(One-Half)	(One-Quarter)	(Three-Eighths)

The top number says how many slices we have. 
The bottom number says how many equal slices the whole pizza was cut into.

Some fractions may look different, but are really the same, for example: 

4/8	=	2/4	=	1/2
(Four-Eighths)		(Two-Quarters)		(One-Half)
	=		=

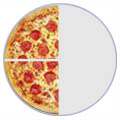It is usually best to show an answer using the simplest fraction ( ¹/₂ in this case ). That is calledSimplifying, or Reducing the Fraction

Numerator / Denominator

We call the top number the Numerator, it is the number of parts we have.
We call the bottom number the Denominator, it is the number of parts the whole is divided into.

NumeratorDenominator

You just have to remember those names! (If you forget just think "Down"-ominator)

Adding Fractions

It is easy to add fractions with the same denominator (same bottom number):

1/4	+	1/4	=	2/4	=	1/2
(One-Quarter)		(One-Quarter)		(Two-Quarters)		(One-Half)
	+		=		=

One-quarter plus one-quarter equals two-quarters, equals one-half

Another example:

5/8	+	1/8	=	6/8	=	3/4
	+		=		=

Five-eighths plus one-eighth equals six-eighths, equals three-quarters

Adding Fractions with Different Denominators

But what about when the denominators (the bottom numbers) are not the same? 

3/8	+	1/4	=	?
	+		=

Three-eighths plus one-quarter equals ... what?

We must somehow make the denominators the same.

In this case it is easy, because we know that ¹/₄ is the same as ²/₈ :

3/8	+	2/8	=	5/8
	+		=

Three-eighths plus two-eighths equals five-eighths